Logic Behind Analysis of Variance (ANOVA)

 Logic Behind Analysis of Variance (ANOVA)

## Logic Behind Analysis of Variance (ANOVA)

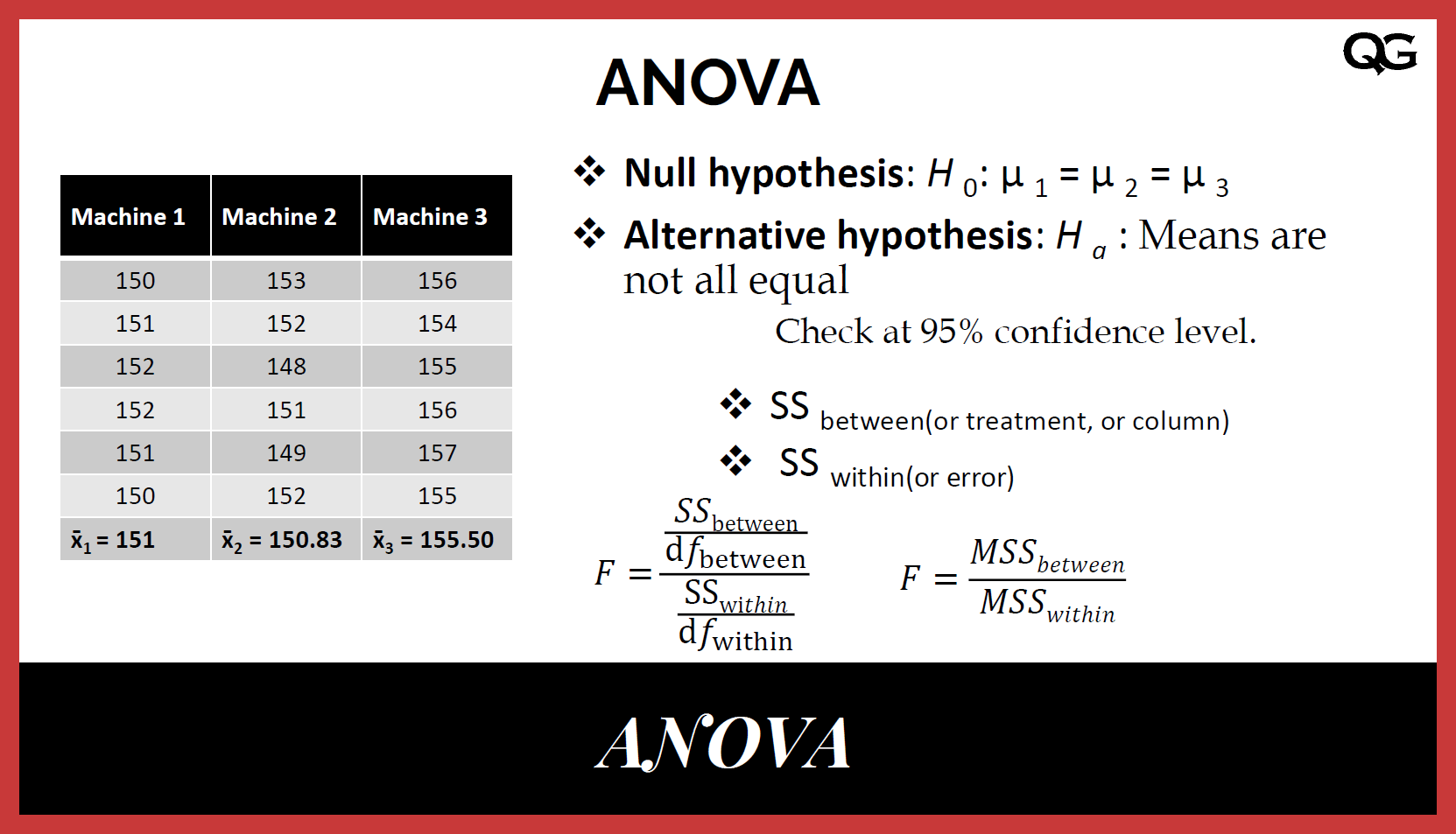
**Analysis of Variance (ANOVA)** is a statistical method used to test differences between two or more group means. The fundamental logic behind ANOVA is to assess whether the variability in the data can be attributed to the differences between the group means or if it is simply due to random chance.

### Key Concepts:

1. **Total Variability**: ANOVA partitions the total variability observed in the data into two components:

   - **Between-Group Variability**: This reflects the variation due to the interaction between the different groups being compared. It measures how much the group means differ from the overall mean.

   - **Within-Group Variability**: This reflects the variation within each group. It measures how much individual observations within each group differ from their respective group mean.

2. **F-Ratio**: ANOVA computes an F-ratio, which is the ratio of the variance between groups to the variance within groups. A higher F-ratio suggests that the variability between group means is greater than the variability within groups, indicating a significant difference among the group means.

3. **Hypothesis Testing**: The null hypothesis (H0) states that all group means are equal, while the alternative hypothesis (H1) states that at least one group mean is different. ANOVA tests these hypotheses by analyzing the F-ratio and determining the associated p-value.

## Differences Between ANOVA and T-Test

### Key Differences:

- **Number of Groups**: The most significant difference is that a t-test is used to compare the means of two groups, while ANOVA is used to compare the means of three or more groups.

  

- **Statistical Output**: A t-test produces a t-statistic and a corresponding p-value, while ANOVA produces an F-statistic and a p-value.

- **Complexity**: ANOVA can handle more complex experimental designs, including factorial designs, where multiple independent variables are analyzed simultaneously.

### When to Use Each:

- **T-Test**: Use when comparing the means of two groups (e.g., comparing test scores between two different teaching methods).

  

- **ANOVA**: Use when comparing the means of three or more groups (e.g., comparing test scores among three different teaching methods).

## Application of ANOVA in Sociological Research

ANOVA is particularly useful in sociological research when examining the effects of categorical independent variables on continuous dependent variables. Here are some situations where ANOVA would be appropriate:

1. **Comparing Group Differences**: When a researcher wants to compare the impact of different social programs on participants' outcomes (e.g., income levels across different training programs).

2. **Assessing Treatment Effects**: In experimental designs, ANOVA can be used to evaluate the effectiveness of multiple interventions (e.g., comparing the effectiveness of different community outreach strategies on public health).

3. **Analyzing Survey Data**: When analyzing survey responses from different demographic groups (e.g., comparing satisfaction levels across various age groups or income levels).

In summary, ANOVA is a powerful statistical tool that helps researchers determine whether significant differences exist among group means, making it essential for analyzing complex social phenomena in sociological research. It provides insights that can inform policy decisions and enhance understanding of social dynamics.

Citations:

[1] https://www.wallstreetmojo.com/anova-vs-t-test/

[2] https://keydifferences.com/difference-between-t-test-and-anova.html

[3] https://testbook.com/key-differences/difference-between-t-test-and-anova

[4] https://www.voxco.com/blog/anova-vs-t-test-with-a-comparison-chart/

[5] https://www.raybiotech.com/learning-center/t-test-anova/

[6] https://www.youtube.com/watch?v=4WtnVOAefPo

[7] https://www.ncbi.nlm.nih.gov/pmc/articles/PMC6813708/

[8] https://www.reddit.com/r/statistics/comments/12u4zgj/q_why_run_a_ttest_instead_of_an_oneway_anova/

Importance of Measures of Central Tendency and Dispersion in Sociological Analysis

 Importance of Measures of Central Tendency and Dispersion in Sociological Analysis

 ## Importance of Measures of Central Tendency and Dispersion in Sociological Analysis

In sociological research, summarizing and understanding the characteristics of data is crucial for drawing meaningful conclusions. Measures of central tendency and measures of dispersion play a vital role in this process by providing concise yet informative statistics that capture the essence of a dataset. Let's explore how these measures help in sociological analysis:

### Measures of Central Tendency

**Mean, Median, and Mode**:

- **Mean**: The arithmetic average, calculated by summing all values and dividing by the number of observations. It represents the central point and is useful for understanding the overall level of a variable[1][4].

- **Median**: The middle value when data is ordered from least to greatest. It is less affected by outliers and skewed distributions, providing a more robust measure of central tendency[1][4].

- **Mode**: The value that occurs most frequently in the dataset. It can reveal the most common response in survey research or the typical value for a variable[1][4].

These measures help sociologists summarize the central tendency of a variable, identify patterns, and make comparisons between groups or time periods[1][2]. For example, comparing the median income of different social classes can uncover disparities in wealth distribution[1].

### Measures of Dispersion

**Range, Variance, and Standard Deviation**:

- **Range**: The difference between the highest and lowest values in a dataset. It provides a simple measure of the spread of data[5].

- **Variance**: A measure of the average squared deviation from the mean. It quantifies the overall variability in the dataset[5].

- **Standard Deviation**: The square root of the variance. It represents the average distance of values from the mean and is more interpretable than variance[5].

Measures of dispersion complement central tendency by providing insights into the spread and variability of data. They help identify outliers, assess the consistency of a variable, and determine the reliability of central tendency measures[2][5]. For instance, a high standard deviation indicates that values are spread out from the mean, suggesting greater variability in the data[5].

### Importance in Sociological Analysis

1. **Data Summarization**: Central tendency and dispersion measures condense large datasets into a few representative values, facilitating data interpretation and communication of research findings[1][2].

2. **Comparison and Analysis**: These measures enable sociologists to compare variables, identify patterns, and analyze trends within and across different groups or time periods[1][2].

3. **Hypothesis Testing**: Central tendency and dispersion statistics are essential for formulating and testing hypotheses. For example, researchers can compare the mean values of two groups to determine if there are significant differences[1][2].

4. **Identifying Outliers**: Measures of dispersion, particularly the range and standard deviation, help identify extreme values that may significantly impact the interpretation of research findings[1][4].

5. **Assessing Data Quality**: Analyzing the central tendency and variability of data can reveal potential errors, inconsistencies, or biases in data collection and sampling[2].

By employing measures of central tendency and dispersion, sociologists can gain a comprehensive understanding of their data, draw more accurate conclusions, and communicate their findings effectively to inform social policies and interventions.

Citations:

[1] https://easysociology.com/research-methods/central-tendency-in-research-an-outline-and-explanation-in-sociology/

[2] https://www.alooba.com/skills/concepts/statistics/measures-of-central-tendency/

[3] https://www.wiley.com/en-us/Basic%2BStatistics%2Bfor%2BSocial%2BResearch-p-9781118234150

[4] https://easysociology.com/research-methods/understanding-a-univariate-analysis/

[5] https://statisticsbyjim.com/basics/measures-central-tendency-mean-median-mode/

[6] https://www.abs.gov.au/statistics/understanding-statistics/statistical-terms-and-concepts/measures-central-tendency

[7] https://revisesociology.com/2023/10/10/univariate-analysis-in-quantitative-social-research/

[8] https://bookdown.org/tomholbrook12/bookdown-demo/measures-of-central-tendency.html

Analysis of Variance (ANOVA)

Analysis of Variance (ANOVA)

 

### Unit V: Analysis of Variance (ANOVA)

#### A. **The Logic of Analysis of Variance**

Analysis of Variance (ANOVA) is a statistical technique used to determine whether there are significant differences between the means of three or more groups. The key logic behind ANOVA is to test the hypothesis that all group means are equal, versus the alternative hypothesis that at least one group mean is different. 

ANOVA compares the variance within each group to the variance between the groups:

- **Within-group variance** measures how much individuals in the same group differ from the group mean.

- **Between-group variance** measures how much the group means differ from the overall mean.

If the between-group variance is significantly larger than the within-group variance, it suggests that the groups are not all the same, leading to the rejection of the null hypothesis.

The F-ratio is used in ANOVA to compare these variances:

\[

F = \frac{\text{Between-group variance}}{\text{Within-group variance}}

\]

If the F-ratio is large, it suggests that there is a significant difference between group means.

---

#### B. **Analysis of Variance**

ANOVA can be conducted for different types of data:

- **One-Way ANOVA**: Used when comparing the means of three or more independent groups on one factor. For example, you might compare the academic performance (measured by test scores) of students from three different educational methods.

  

  Steps in One-Way ANOVA:

  1. Calculate the **total variance** (the variance of all observations).

  2. Break down the total variance into **between-group variance** and **within-group variance**.

  3. Compute the **F-ratio**.

  4. Compare the F-ratio to a critical value from the F-distribution table, which depends on the number of groups and sample sizes. If the calculated F-ratio is larger than the critical value, the null hypothesis (that all group means are equal) is rejected.

- **Two-Way ANOVA**: Used when there are two independent variables, allowing the researcher to assess not only the main effects of each variable but also the interaction effect between the two variables. For instance, you might examine the effects of both gender and study method on academic performance.

---

#### C. **Multiple Comparison of Means**

After conducting ANOVA, if the null hypothesis is rejected, it indicates that at least one group mean is different, but it doesn’t specify which groups are significantly different. To determine which specific group means differ from each other, **multiple comparison tests** (also called post hoc tests) are used. Common methods include:

- **Tukey’s Honestly Significant Difference (HSD)**: Compares all possible pairs of means to identify which ones are significantly different.

  

- **Bonferroni Correction**: Adjusts the significance level to account for multiple comparisons, reducing the chance of Type I errors (false positives).

- **Scheffé’s Test**: A more conservative post hoc test, especially useful when comparing all possible contrasts between means, not just pairwise comparisons.

These tests help provide a clearer picture of where the significant differences lie between the groups, beyond simply knowing that differences exist.

---

### **Readings** for this Unit:

1. **Levin and Fox**. (1969). *Analysis of Variance* (Chapter 8, pp. 283-308): This chapter provides an overview of the theory and application of ANOVA, focusing on how to conduct the analysis and interpret the results.

2. **Blalock, H.M.** (1969). *Analysis of Variance* (Chapter 16, pp. 317-360): This reading delves deeper into the mathematical foundation of ANOVA, offering a more comprehensive understanding of the statistical principles involved.

These readings will give you a solid foundation in understanding and applying ANOVA in sociological research, particularly when comparing group means. Let me know if you need further elaboration on any specific point!

Analysis of Interval- and Ratio-scale Data

Analysis of Interval- and Ratio-scale Data

 

### Unit IV: Analysis of Interval- and Ratio-scale Data

#### A. **Rationale**

Interval- and ratio-scale data allow for more sophisticated statistical analyses because both scales measure continuous variables. Interval data has meaningful intervals between values, but no true zero point (e.g., temperature in Celsius), while ratio data has a true zero (e.g., income, age). The rationale for analyzing such data is to gain deeper insights into relationships, patterns, and trends, making it possible to perform tests of significance and assess the strength and nature of relationships between variables. This allows researchers to make more precise and reliable inferences about populations.

---

#### B. **Univariate Data Analysis: One-Sample Z, t, and F Tests**

- **Z Test**: A statistical test used to determine whether the mean of a population is significantly different from a hypothesized value when the population variance is known and the sample size is large (n > 30).

  - Formula: 

    \[

    Z = \frac{\bar{X} - \mu}{\sigma / \sqrt{n}}

    \]

    Where:

    - \(\bar{X}\) = Sample mean

    - \(\mu\) = Population mean

    - \(\sigma\) = Population standard deviation

    - \(n\) = Sample size

- **t-Test**: Used when the population variance is unknown and the sample size is small (n < 30). It tests whether the sample mean is significantly different from a hypothesized population mean.

  - Formula:

    \[

    t = \frac{\bar{X} - \mu}{s / \sqrt{n}}

    \]

    Where:

    - \(s\) = Sample standard deviation (used instead of population standard deviation).

- **F Test**: Used to compare the variances of two populations or assess whether multiple group means differ significantly (ANOVA). This test is critical for understanding whether variability between groups is due to chance or a real difference.

---

#### C. **Bivariate Data Analysis**

- **Two-Way Frequency Table**: Similar to nominal data analysis, but in interval/ratio data, the emphasis is more on measuring the strength of the relationship between variables.

- **Scatter Diagram**: A graphical representation that plots two variables on a Cartesian plane. It helps in visualizing the relationship between two interval or ratio variables. The pattern in the scatter diagram provides clues about the direction and strength of the relationship.

- **Correlation Coefficient**: Measures the strength and direction of the relationship between two variables. The most common is **Pearson’s r**, which ranges from -1 to 1. A value close to 1 or -1 indicates a strong relationship, while a value near 0 indicates a weak or no relationship.

  - Formula for Pearson's r:

    \[

    r = \frac{n(\sum xy) - (\sum x)(\sum y)}{\sqrt{[n\sum x^2 - (\sum x)^2][n\sum y^2 - (\sum y)^2]}}

    \]

- **Simple Linear Regression**: A method for predicting the value of a dependent variable based on the value of an independent variable. It establishes a linear relationship between two variables.

  - Formula: 

    \[

    Y = a + bX

    \]

    Where:

    - \(Y\) = Dependent variable

    - \(X\) = Independent variable

    - \(a\) = Intercept

    - \(b\) = Slope (rate of change).

- **Two-Sample Z, t, and F Tests**: These are extensions of the one-sample tests, used when comparing two independent groups:

  - **Two-sample Z Test**: Compares the means of two independent samples when the population variances are known.

  - **Two-sample t-Test**: Used when population variances are unknown, and it tests whether two sample means differ significantly.

  - **Two-sample F Test**: Compares the variances of two independent samples.

- **Significance Tests of Correlation and Regression Coefficients**: These tests determine whether the observed correlation or regression coefficients are statistically significant. The hypothesis test checks if the correlation or slope coefficient is significantly different from zero, indicating a meaningful relationship between the variables.

---

#### D. **Interpretation**

The interpretation of these analyses involves understanding the meaning of the statistical output and its implications. For example:

- In correlation analysis, you interpret the direction (positive or negative) and strength of the relationship.

- In regression analysis, the slope coefficient (\(b\)) indicates the rate of change in the dependent variable for each unit change in the independent variable.

- In significance tests, p-values are used to determine whether the results are statistically significant. A p-value less than 0.05 typically indicates that the relationship or difference is not due to random chance.

---

#### E. **Inference**

Inferences from interval and ratio data analysis help researchers generalize their findings from a sample to the larger population. These tests allow you to make informed conclusions, such as predicting outcomes (e.g., predicting income based on education level), or understanding the strength and nature of relationships between variables in the population. Confidence intervals and hypothesis testing are essential for making these inferences reliable.

---

### **Readings** for this Unit:

1. **Blalock, H.M.** (1969). *Interval Scales: Frequency distribution and graphic presentation* (Chapter 4, pp. 41-54): This chapter covers the basics of summarizing interval-scale data using frequency distributions and visual methods like graphs.

2. **Blalock, H.M.** (1969). *Interval Scales: Measures of Central Tendency* (Chapter 5, pp. 55-76): This reading focuses on the measures of central tendency (mean, median, mode) for interval data.

3. **Blalock, H.M.** (1969). *Two Samples Test: Difference of Means and Proportions* (Chapter 13, pp. 219-242): This chapter explains how to test for significant differences between two samples.

4. **Levin and Fox**, *Elementary Statistics in Social Research*, Chapter 7: "Testing Differences between Means" (pp. 235-268): This reading explains various methods for testing mean differences between groups using z, t, and F tests.

5. **Blalock, H.M.** (1969). *Correlation and Regression* (Chapter 17, pp. 361-396): This chapter provides an in-depth understanding of correlation and regression analysis, crucial for analyzing interval and ratio data.

6. **Levin and Fox**, *Elementary Statistics in Social Research*, Chapters 10 and 11 (pp. 345-392): These chapters further elaborate on correlation and regression analysis, including testing for significance of relationships and interpreting regression coefficients.

These readings will guide you through the theoretical and practical aspects of analyzing interval and ratio-scale data in sociological research. Let me know if you'd like to explore any topic in more detail!

Analysis of Nominal-Scale Data

Analysis of Nominal-Scale Data

 

### Unit II: Analysis of Nominal-Scale Data

#### A. **Rationale**

Nominal-scale data refers to data that is categorized without any quantitative value or inherent ranking between the categories. These variables represent distinct groups or types, such as gender, ethnicity, religion, or political affiliation. The key rationale for analyzing nominal data is to summarize and compare proportions or frequencies within different categories, as well as to assess relationships between these categories. Since nominal data does not involve a hierarchy or order, only frequency-based analyses are suitable for such data.

Nominal data is often visualized using bar charts or pie charts to show proportions, and it is analyzed using techniques such as frequency tables and contingency tables to explore relationships between variables.

---

#### B. **Univariate Data Analysis: One-Way Frequency Table**

A **one-way frequency table** is used in univariate analysis (the analysis of a single variable) to display the number of occurrences for each category within a nominal variable. This helps in summarizing how often each category appears in a dataset.

For example, if you are analyzing a dataset on political affiliation with categories such as Democrat, Republican, and Independent, a one-way frequency table would display the count of respondents in each category:

| Political Affiliation | Frequency |

|-----------------------|-----------|

| Democrat              | 100       |

| Republican            | 120       |

| Independent           | 80        |

This table provides a clear, simple representation of how the data is distributed across categories.

---

#### C. **Bivariate Data Analysis: Two-Way Frequency Table and Chi-Square Test**

**Two-Way Frequency Table (Contingency Table)**:

A two-way frequency table, also known as a **contingency table**, is used to explore the relationship between two nominal variables. It shows how frequently each combination of categories occurs. For example, a contingency table might compare **political affiliation** with **gender**:

|                | Democrat | Republican | Independent | Total |

|----------------|----------|------------|-------------|-------|

| Male           | 50       | 70         | 30          | 150   |

| Female         | 50       | 50         | 50          | 150   |

| Total          | 100      | 120        | 80          | 300   |

This table can help sociologists assess whether there is an association between gender and political affiliation.

**Chi-Square Test**:

The chi-square test is a statistical test used to determine whether there is a significant association between two nominal variables. It compares the observed frequencies in the contingency table to the expected frequencies (what would occur if there were no association between the variables).

The formula for the chi-square statistic (χ²) is:

\[

\chi^2 = \sum \frac{(O - E)^2}{E}

\]

Where:

- **O** = Observed frequency

- **E** = Expected frequency (calculated under the assumption of no relationship between the variables)

If the calculated chi-square value exceeds a certain threshold (based on the degrees of freedom and significance level), the null hypothesis (no relationship between the variables) is rejected, indicating that a significant association exists.

---

#### D. **Level of Significance (Measures of Strength of Relationship)**

In hypothesis testing, the **level of significance** (denoted by **α**) is the threshold for determining whether to reject the null hypothesis. Typically, α is set at 0.05, meaning that there is a 5% risk of rejecting the null hypothesis when it is actually true (a Type I error).

- **P-value**: The p-value indicates the probability of observing the test results under the assumption that the null hypothesis is true. If the p-value is less than the level of significance (e.g., p < 0.05), the null hypothesis is rejected.

- **Cramér's V**: This is a measure of the strength of association between two nominal variables. Cramér's V ranges from 0 (no association) to 1 (perfect association). It is derived from the chi-square statistic and accounts for the size of the table.

---

#### E. **Interpretation**

The interpretation of results from chi-square tests or frequency tables involves determining whether there is a statistically significant relationship between variables. If the chi-square test shows significance (p < 0.05), it indicates that the observed relationship between the variables is unlikely to have occurred by chance.

- In the context of a two-way table, the interpretation involves looking at whether the distribution across categories deviates from what would be expected under the assumption of no association.

- In addition, the strength of the relationship (using Cramér's V) can help in determining whether the relationship, even if significant, is weak or strong.

For example, in the political affiliation and gender analysis, if the chi-square test is significant, it may suggest that gender is related to political affiliation in the sample.

---

#### F. **Inference**

Inference in nominal-scale data analysis refers to making generalizations about a population based on the analysis of a sample. After conducting tests like chi-square, sociologists can infer whether the relationships observed in the sample likely hold true for the larger population. This is done while acknowledging the limitations of the data, including sample size, potential biases, and random error.

For example, if the chi-square test reveals a significant relationship between gender and political affiliation in the sample, a researcher might infer that gender plays a role in political affiliation in the broader population, assuming the sample is representative.

---

### **Readings** for this Unit:

1. **Blalock, H.M.** (1969). *Nominal Scales: Proportions, Percentages, and Ratios* (Chapter 3, pp. 31-40): This reading focuses on the application of proportions, percentages, and ratios in the analysis of nominal data, providing a detailed understanding of how these tools can summarize nominal-scale data effectively.

2. **Blalock, H.M.** (1969). *Nominal Scales: Contingency Problems* (Chapter 15, pp. 275-316): This chapter delves into the challenges of analyzing relationships between nominal variables using contingency tables and offers solutions for accurately interpreting contingency problems in sociological research.

These readings will deepen your understanding of nominal-scale data analysis and its application in sociological research. Let me know if you'd like further elaboration on any of these topics!